Introduction
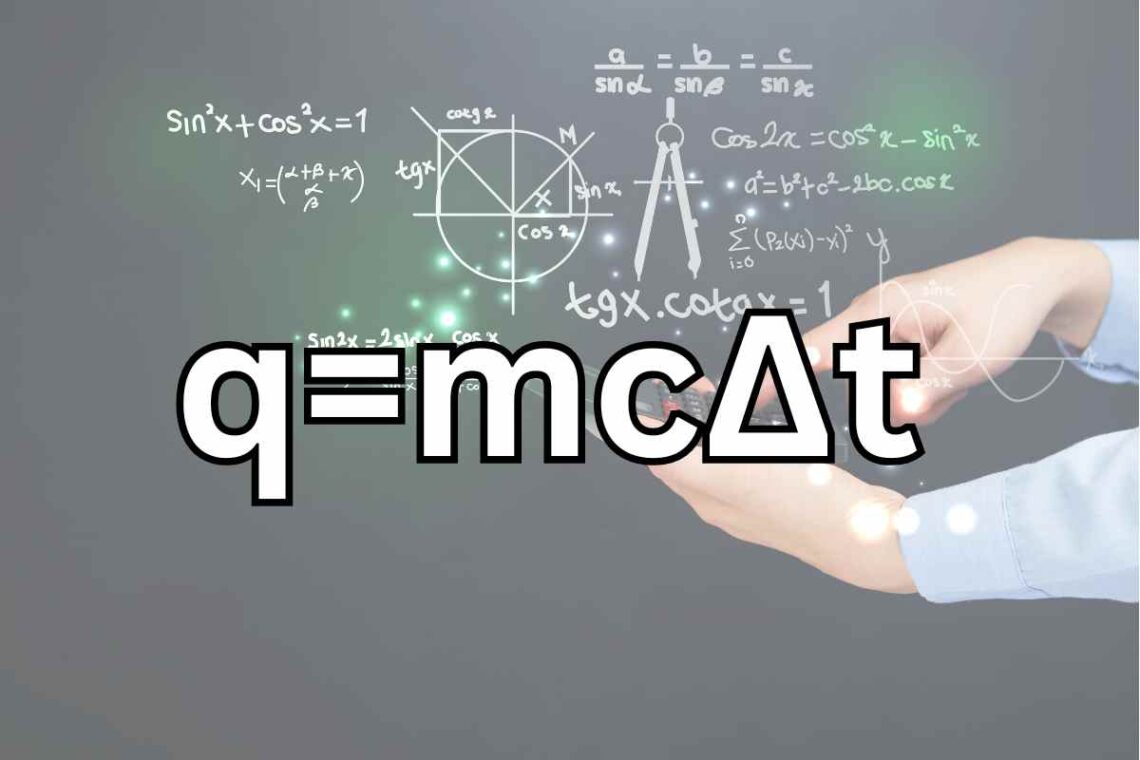
The equation “q=mc∆t” is a fundamental concept in physics used to describe heat transfer in various systems. In this article, we will delve into the intricacies of this equation, understand its historical significance, explore its practical applications, and shed light on its importance in the realms of thermodynamics and chemistry. Join us as we demystify the equation “q=mc∆t” and its role in the scientific world.
Understanding the Equation
The equation “q=mc∆t” is composed of several variables, each with its unique significance. “q” represents the heat transfer, “m” is the mass of the substance involved, “c” stands for the specific heat capacity of the material, and “∆t” signifies the temperature change. These variables work together to quantify the heat transferred in a given scenario.
Historical Context
The origins of “q=mc∆t” date back to the early days of modern physics. Prominent scientists like Antoine Lavoisier, Joseph Black, and James Joule played pivotal roles in developing this equation. Lavoisier’s work on the conservation of mass and Black’s experiments on heat laid the foundation for our understanding of heat transfer. James Joule’s experiments in the 19th century further solidified the equation’s importance in the field of thermodynamics.
Practical Applications
The equation “q=mc∆t” finds applications in various real-world scenarios. It determines the heat generated or absorbed in chemical reactions, making it crucial in calorimetry. Additionally, it is employed in industries like engineering and metallurgy to calculate heat transfer in various processes.
The Relationship Between Heat and Temperature
One of the critical aspects of “q=mc∆t” is its connection between heat and temperature. When a substance undergoes a temperature change, heat is either absorbed or released. Understanding this relationship is vital in many scientific experiments and industrial processes.
Significance in Thermodynamics
The equation “q=mc∆t” is a cornerstone of the first law of thermodynamics, also known as the law of energy conservation. It asserts that energy cannot be created or destroyed, only transformed. This equation plays a vital role in understanding how energy is conserved in various physical and chemical processes.
Calculations and Units
Performing calculations using “q=mc∆t” requires attention to units of measurement. The mass must be measured in kilograms, particular heat capacity expressed in joules per gramme at a temperature of C and the temperature change in degrees Celsius. Careful unit conversions are often necessary to ensure accurate calculations.
Limitations and Assumptions
While “q=mc∆t” is a powerful tool, it has certain limitations and assumptions. For example, it assumes that specific heat capacity remains constant over the entire temperature range, which is not always true. Understanding these limitations is crucial for accurate application.
Real-World Examples
In the real world, “q=mc∆t” is applied in various experiments and industries. Chemistry helps determine the heat released or absorbed during a chemical reaction. In engineering, it’s essential for understanding heat transfer in multiple processes, such as in the cooling systems of engines.
Importance in Chemistry
Chemists use “q=mc∆t” extensively, especially in calorimetry. This branch of chemistry involves measuring heat changes in chemical reactions. By using this equation, chemists can determine the energy changes that occur during responses, providing valuable insights into the thermodynamics of chemical processes.
Challenges and Misconceptions
Applying “q=mc∆t” correctly can be challenging, and common misconceptions are associated with it. Some may overlook the need for accurate measurement and unit conversions, leading to inaccurate results. Understanding these challenges and misconceptions is essential for accurate scientific analysis.
Case Studies
To illustrate the practical importance of “q=mc∆t,” let’s explore a few case studies. We will look at how this equation was pivotal in understanding heat transfer in specific experiments, from calorimetry in chemistry labs to the design of efficient cooling systems in industrial settings.
Theoretical and Experimental Insights
One of the fascinating aspects of “q=mc∆t” is its ability to predict heat transfer in theoretical scenarios and compare these predictions with experimental results. Over the years, countless experiments have confirmed the accuracy of this equation, further establishing its importance in scientific research.
The Future of “q=mc∆t”
As science and technology advance, so does the understanding and application of “q=mc∆t.” This equation will likely evolve, finding new applications in emerging technologies and scientific research. Its relevance in our ever-changing world of science remains unwavering.
Conclusion
In conclusion, “q=mc∆t” is not just a mathematical equation; it is a cornerstone of our understanding of heat transfer and energy conservation. From its historical roots to its practical applications in various fields, this equation continues to shape how we view the world of physics and chemistry.
5 Unique FAQs
Q1: What is the significance of “q=mc∆t” in everyday life?
“q=mc∆t” helps us understand how heat is transferred and how energy is conserved in everyday processes, from cooking to the operation of engines.
Q2: How do scientists ensure the accuracy of calculations using “q=mc∆t”?
Scientists ensure accuracy by precisely measuring mass, specific heat capacity, and temperature changes, as well as converting units correctly.
Q3: Can “q=mc∆t” be applied to biological systems?
Yes, “q=mc∆t” can be applied to biological systems to measure heat transfer in various biological processes and reactions.
Q4: What are some practical examples of “q=mc∆t” in industry?
Industries use this equation in designing efficient cooling systems, managing heat in metallurgy, and determining heat changes in chemical processes.
Q5: How has “q=mc∆t” contributed to developing renewable energy technologies?
“q=mc∆t” plays a role in understanding energy transfer in renewable energy systems, such as solar panels and geothermal heat pumps, aiding their optimization and efficiency.